Mechanics Behind Biological Nanotechnology

Let us make an in-depth study of the mechanics behind biological nanotechnology. The below given article will help you to learn about the following things:- 1. Science at the Biology—Nanotechnology Interface 2. Scales at the Bio-Nano Interface 3. Modelling at the Nano-Bio Interface 4. Nature’s Nanotechnology: Viruses as an Example and 5. Concluding Remarks.

Science at the Biology—Nanotechnology Interface:

Biological Nanotechnology:

Though the innovations leading to the adop­tion of the expression ‘nanotechnology’ are indeed impressive, the perspective of this article is that one need only look inward to the way that our muscles move, to the way how we digest and synthesize molecules of dazzling complexity, to the way in which we think the thoughts that permit us to fill the shelves of libraries with scientific journals to realize that the greatest nanotechnology of all is that which is revealed in the living world.

That is, one of the central thrusts is the idea that the microscopic workings of life offer an inspir­ing vision of nanotechnology. The ambition of the present section is to provide several cursory examples of the Nano technological marvels that power the living realm.

Self-Assembly as Biological Nanotechnology:

One of the most intriguing Nano technological tricks of the living world is the central role played by self-assembly in such systems. Whether we consider the spontaneous assem­bly of viruses, either in test tubes or in the in­terior of an infected cell or the fusion of one membrane-bound region to another through vesicle fusion, spontaneous formation of functional ‘materials’ is a key part of the bio­logical repertoire.

To be more concrete, we note that self-assembly in biological systems takes place in a number of different guises. First, the self-assembly of linear assemblies is a key part of the cytoskeletal assembly pro­cess with G-action associating to form actin filaments and, similarly, tubulin monomers joining to form microtubules.

This is also the process that is used by certain bacteria such as Listeria for locomotion. This same type of process is taken to the next level of sophisti­cation in simple viruses such as tobacco virus that involve not only the assembly of protein monomers, but also the genetic message in the form of long RNA molecules.

A second broad class of self-assembly processes is asso­ciated with the formation of containers such as liposomes or viral capsids. In the case of liposomes, lipid molecules such as phospatidycholine spontaneously organize in a way that sequesters the hydrophobic tails from the surrounding water. Similarly, protein subunits spontaneously assemble to form viral capsids.

These structures play a variety of roles in the biological realm, from serving as containers for different macromolecules to providing for concentration gradients of small ions that are maintained and utilized by molecular motors such as ATP synthase.

Beyond the simplistic description of self- assembly advanced above, a second key fea­ture of biological self-assembly must also be considered. In particular, a variety of self-assembly processes in the biological realm are templated (or coded).

As is well-known, protein are a hugely versatile class of mol­ecules all based upon the same fundamental building blocks. Interestingly, the enormous diversity of protein action is founded upon 20 distinct amino acid building blocks, and the template for assembling a given protein is carried in the form of messenger RNA, which is then read and used as the basis for protein synthesis by the ribosome. One of the most exciting developments in modern nanotech­nology is the attempt to exploit templated self-assembly processes for the purposes of creating new materials.

Molecular Motors as Biological Nanotechnology:

One of the hallmarks of life is change and motion. At the cellular level, such motion is affected through a dizzying variety of mecha­nisms, most of which when viewed at the molecular level are seen to be the result of the action of molecular motors. For example, muscle contraction reflects the coherent ac­tion of huge numbers of myosin motor mol­ecules as they march along actin filaments.

Similarly, the motion of certain bacteria can be traced to the rotation of a rotary motor embedded in the cell wall, which is attached to filaments known as flagella. In a similar vein, just as our modern society is replete with examples of systems aimed at allowing for communication and transport between widely separated geographic locations, so, too, has the living world has to answer these same challenges.

This article aimed at making estimates of various scales and processes in nanotech­nology, one of the key mechanisms for com­munication and transport is diffusion. That is, a chemical concentration at one place can make its presence known at a distance x with a characteristics time, t diffusive = x²/2D, where D is the diffusion constant.

There are many cellular processes that cannot wait as long as t diffusion. As a result, a host of molecular mo­tors and an associated transport system (the elements of the cytoskeleton) permit active transport. For example, kinesin is a motor molecule that transports material along long, relatively rigid polymeric assemblies known as microtubules.

Even more incredible are rotary machines like those associated with ATP synthase and bacterial flagella. ATP syn­thase is a membrane-embedded machine that rotates and, in so doing, synthesizes new ATP molecules (adenosine triphosphate, energy currency of the cell).

Similarly, the bacterial flagellar motor rotates with the result that the attached bacterial flagellum rotates and there­by induces motion of the cell. The exquisite details of the construction of these devices are themselves breath-taking. The rotary mo­tors in bacteria are constructed form several components that are much like a rotor and stator and perform periodic motions by de­riving energy from the flow of protons across a membrane.

These rotary motors are very powerful, as evidenced by F1-ATP synthase, which can generate a torque large enough to rotate a molecule of action 100 times its own length. In addition, bacterial motors have another layer of sophistication in that stim­uli from the external environment, which are sensed by the bacteria through the pores on its membrane, can change the direction of rotation of the motor in a process known as chemo-taxis.

Feedback and signal transmis­sion are implemented in engineering devices by means of complex circuitry, whereas in the living would these functions are often accom­plished by means of chemistry and confor­mational changes in large molecules.

All of these molecular machines involve a rich interplay between chemistry, thermo­dynamics, and mechanics. From a structural perspective, most molecular motors are pro­teins with different subunits performing dif­ferent functions. Often there is some region within the motor where chemical energy is derived from the hydrolysis of adenosine triphosphate (ATP).

This chemical energy is then converted to mechanical energy through a conformational change in the protein. These examples serve to call the reader’s atten­tion to the importance and variety of motor molecules found throughout the living world and which almost any sensible definition of nanotechnology would have to include as particularly sophisticated examples.

Molecular Channels and Pumps as Biological Nanotechnology:

From a structural perspective, one of the most intriguing features of cellular systems is their division into a number of separate membrane-bound compartments. We have already touched upon this compartmentalization in the context of self-assembly.

The presence of such compartments, often marked by large concentration gradients with respect to the surrounding medium, hints at another Nano technological wonder of the living world, namely, the presence of a wide range of trans-membrane channels that medi­ate the exchange of material between these different compartments.

Certain passive ver­sions of these channels are gated by various mechanisms such as the arrival of signaling molecules or tension in the membrane within which they are found. Once the channel is in the open state, ions pass through passively, by diffusion.

Active versions of ion channels that transfer ions such as Na⁺ and Ca²⁺ are simi­larly critical for the functioning of a cell, both in the case of unicellular and multicellular or­ganisms. For example, in the case of Na⁺ ions, typical concentrations within the cell can be as much as a factor of 10-20 less than those in the extracellular milieu.

Such concentration gradients imply the need for sophisticated ac­tive ‘devices’ which can do work against such gradients. One of the most remarkable of ma­chines of this type is the Na⁺-K⁺ pump. This machine is powered by hydrolysis of ATP (i.e., the consumption of ATP fuel), and it can pump ions up a potential gradient.

In partic­ular, this pump hydrolyses ATP and pumps Na⁺ ions out of the cell against a very steep concentration gradient while pumping K⁺ ions in again against a steep gradient. The point of this article has been to illustrate the first of several perspectives that we will bring to bear on the question of the biology nanotechnology interface.

Thus far, we have noted that nature is replete with examples of macromolecules and macromolecular assemblies that perform Nano technological tasks and in this capacity serve as examples of biological nanotechnology. Next, we wish to examine the ways in which biological phenomena can inspire nanotechnology itself.

Biologically Inspired Nanotechnology:

Biological systems are Nano technologically relevant for several reasons. First, as shown earlier, the living world is full of examples of Nano technological devices. However, a sec­ond key point is that nanotechnology has been driven and inspired by the example of biological systems and the need (for example, in medicine) to influence biological systems at the scale of a single cell.

In addition, pre­liminary steps have been taken to harness the nanotechnology of biological systems and use it to perform useful functions. One compel­ling example of a proof-of-principle—bio­logically inspired device emerged from work aimed at exploring the function of ATP synthase, the rotary device already described above. Fluorescently labeled filamentous proteins from the cytoskeleton, known as actin, were attached to the putative rotary com­ponent of the F1 subunit. The point of this exercise is that such filaments are observable using light microscopy.

It was then observed that the long actin filament rotated like a pro­peller when the ATP synthase performed ATP hydrolysis. A second example, also involving ATP synthase, is suggested by a set of experi­ments as illustrated schematically in [Fig. 4.1].

Two different protein machines are ‘reconsti­tuted’ in an artificial membrane bound region known as a liposome. One of these devices is known as bacteriorhodopsin and has the ca­pacity to pump hydrogen ions when it is ex­posed to light.

Since both the bacteriohodopsin and ATP synthase are embedded in the same membrane, the ATP synthase can then exploit the light-induced proton gradient to perform ATP synthesis. Again, these experi­ments were undertaken not for their role as possible devices, but rather to probe the na­ture of various molecular machines.

Neverthe­less, we view them as a provocative demonstration of both the manipulation and use of such machines in artificial environments. As such, they provided an inspiring vision of the possibilities for biologically inspired nano­technology.

Another fascinating example of biologically inspired nanotechnology is that of bio-functionalized cantilevers. A typi­cal example is provided by the experiments demonstrating the use of a bio-functionalized cantilever as a scheme for detecting small concentrations of biologically interesting molecules such as prostate specific antigen and single-stranded DNA. One surface of the cantilever is coated with an antibody, and then it is placed in environments containing different concentrations of the antigen.

The Key Ideas from a Mechanical Perspective are:

(i) The difference in surface energy between the top and bottom surfaces of the cantilever induces spontaneous bending, and

(ii) The binding of molecules of interest to target mol­ecules initially present on the surface leads to surface energy differences and bending that can be detected by optical means. In this way the concentration of the molecules of interest can be measured.

The specificity and sensi­tivity of the method makes it viable for use in the laboratory, as well as for commercial purposes. We return to this example in our discussion of the modelling challenges posed by problems at the interface between biology and nanotechnology.

Nanotechnology and Single Molecule Assays in Biology:

One of the key refrains of the Nano technological era is Feynman’s quip that ‘there is plenty of room at the bottom’. The benefits of min­iaturization are evident at every turn in applications ranging from our cars to our com­puters. Associated with the development of the inspiring new techniques made possible by nanotechnology has been the emergence of a host of scientific opportunities. One of the arenas to benefit from these new tech­niques is biology.

As scientists and engineers have taken the plunge to the Nano technological ‘bottom’ foreseen by Feynmann, oppor­tunities have constantly arisen to manipulate biological systems in ways that were hereto­fore unimagined, culminating in a new era of single molecule biology.

Single molecule experiments have, in fact, presented us with a view of Feynman’s ‘room at the bottom’ as being filled with very complicated machines whose functioning makes life possible. Single molecule assays complement statistical/col­lective studies involving a larger number of molecular actors by revealing the prominent role of fluctuations at the sub-cellular scale. For example, a photo spectrometer measures the optical response of a huge collection of molecules, whereas optical tweezers pull on a single molecule of DNA and enable us to follow the changes in conformation or the breakage of bonds.

In fact, experimental methods are so advanced that it is now pos­sible to manipulate a single molecule even as we watch it on a screen as it jiggles around in different conformations. In what follows, we give several examples of how nanotechnology has reached out to help create single molecule biology and, in the process, has led to the ad­vent of new quantitative opportunities to in­vestigate biological systems.

Atomic-Force Microscopy:

One of the tools that have revolutionized nanotechnol­ogy, in general, and single molecule biology, in particular, is the atomic-force microscope. The AFM has helped create the field of sin­gle molecule force spectroscopy. We note that mechanics has a long tradition of using force-extension data (much like the electrical engineer uses current-voltage data) to probe the inner workings of various materials. It is now possible to apply forces of known mag­nitude on a macromolecule and study how it deforms under the force.

This furnishes structural information and provides insights into the energy landscape the molecule needs to navigate as it undergoes force-induced conformational changes. The energy of de­formation associated with such molecules is primarily determined by weak forces such as hydrogen bonds, van der Waals’ contacts, and gyration effects.

On a more philosophical note, these experiments force us to think in terms of forces and not energy, complement­ing the traditional views held in molecular biology, and they can lead to many new in­sights about the relation between structure and function in proteins, polynucleotides and other macromolecular entities.

The AFM has been used in a wide variety of single molecule experiments on many of the key classes of molecules found in the living world, includ­ing nuclei acids, proteins, and carbohydrates. One fascinating example is the use of atomic force microscopy to examine the mechanical properties of the muscle protein titin, which shows that there is a series of force-extension signatures (increasing force and extension followed by a precipitous load drop) that cor­respond to the unbinding of the individual domains that make up this protein.

Optical Tweezers:

Another instrument that has been used with great success in the realm of biophysics for the purposes of per­forming Nano mechanical measurements on macromolecules and their assemblies is the optical tweezer. While the AFM is relatively stiff and applies large forces (on the order of 100-1,000 pN), optical tweezers are compli­ant and can measure smaller forces (on the order of 0.1-10 pN).

Some of the most inter­esting experiments performed with optical tweezers concern the functioning of molecu­lar motors, which by themselves are marvels of nanotechnology. For example, kinesin is attached to an optically trapped bead and its movement observed along a microtubule. An example of the type of data to emerge from such experiments is shown in Fig. 4.2.

One of the conclusions to emerge from such experiments is that kinesin can exert forces on the order of 5-7 pN before it stalls. Such ex­periments also permit an examination of the effect of changing the concentration of ATP on the functioning of kinesin.

Similar experi­ments have been performed on RNA poly­merase as it advances along DNA to deduce not only the stall force, but also its velocity as a function of the constraining force. Such measurements provide a mechano-chemical basis of biological function and go a long way in revealing connections between chemi­cal kinetics and mechanical processes at the molecular level.

One of the most fascinating examples of the biology-nanotechnology in­terface is that of bacterial viruses, known as bacteriophages. The life cycle of a large class of bacteriophages is characterized by self-assembly processes that lead to the formation of the protein shell of the virus followed by active packaging of the viral DNA within this shell by a motor.

The structure of this so-called ‘viral portal motor’ has been solved using X-ray crystallography. In a recent experiment, scientists used optical tweezers to study the characteristics of the DNA packaging process of the φ -29 bacteriophage. One of the conclu­sions of this experiment is that the motor has to act against an increasing resistive force as more and more of the DNA is packed inside the capsid. From a quantitative perspective, this experiment yields the force and the rate of packing as a function of the fraction of the genome packed.

It is also important to note that bacteriophages are not only an obscure subject of quiet enquiry, but are also the basis of a huge range of cloning products used for doing experiments with recombinant DNA, and more generally, viruses are being ex­plored as the basis of gene therapy.

Our discussion thus far has been aimed at providing a rough overview of the vast land­scape that sits at the interface between biol­ogy and nanotechnology. It is hoped that the few representative case studies set forth above suffice to illustrate our basic thesis, namely, that biological nanotechnology represent nanotechnology at its best.

Challenge of Modelling the Bio-Nano Interface:

As highlighted in the previous two subsec­tions, there have been huge advances at the interface between nanotechnology and biol­ogy. We have argued that there are two dis­tinct representations of the interface between biology and nanotechnology, and each has its own associated set of modelling challenges.

The argument of the present discussion is that another key part of the infrastructure that must attend these developments is that associated with the modelling of these sys­tems. One of the intriguing ways in which modelling at the biology-nanotechnology interface is assuming greater importance is that, with increasing regularity, experimental data on biological systems is of a quantitative character.

As a result, the models that are put forth to greet these experiments must simi­larly be of a quantitative character. As an example of the type of modelling challenges that must be faced in contemplat­ing the types of problems described above, we return to the example of bio functionalized cantilevers as a problem in Nano mechanics.

The basic physics behind the use of bio functionalized cantilevers as sensors is a competi­tion between the elastic bending energy and the surface free energy difference between the upper and lower faces of the cantilever.

The face with the lower free energy per unit area tends to increase its area by bending the can­tilever. The amount of bending, on the other hand, is limited by the elastic energy cost. The utility of this device derives from the fact that the difference in the surface free energy is af­fected by specific binding of target molecules to probe molecules that are initially deposited on one side of the cantilever.

In an experiment, measurement indicate, an upward cantilever deflection when single stranded DNA (ssDNA), up to 20 nucleotides in length, hybridizes with complementary strands of ssDNA, which were deposited ini­tially so as to functionalize the beam. This ef­fect might be attributed to the difference in elastic properties of ssDNA and its double stranded counterpart.

Namely, under physi­ological conditions, ssDNA has a persistence length equal to two nucleotides, while dsDNA is much stiffer (due to hydrogen bonding and base stacking interactions between the two strands) and has a persistence length of 150 nucleotides.

Therefore, deposition of the flex­ible ssDNA molecules initially leads to bend­ing of the cantilever downward due to en-tropic repulsion between the ssDNA chains. After hybridization, rigid dsDNA strands are formed, there is no longer any entropy to be gained by increasing the area of the top sur­face, and the beam bends upwards.

It was demonstrated that this bio-functionalized cantilever is sensitive to ssDNA differing in length by a single nucleotide. While this ex­ample gives a feel for the way in which quan­titative models have been put forth to respond to biologically inspired nanotechnologies, the remainder of the article will emphasize at­tempts to construct Nano mechanical models of biological nanotechnology itself. We turn first to a discussion of the various scales that arise in thinking about the biology-nanotech­nology interface and then conclude with sev­eral modelling examples.

Scales at the Bio-Nano Interface:

Every scientific discipline has a preferred set of units that itself to building intuition concern­ing the system at hand; For example, an as­tronomer thinks of distances between starts in light years, not kilometers. Though most of us have an intuitive sense of the meaning of a kilometer, by the time we add more than six zeros, all intuition is lost. At terrestrial scales, we talk of distances between cities in terms of the flying time or the driving time between them and usually not in terms of hundreds of kilometer.

Similar choices must be faced in the biological setting. For example, a biolo­gist might characterize the complexity of an organism by the size (in kilo base pairs) of the genome and not by the organism’s physi­cal size. The aim of the present section is to highlight some of the key scales and units that reveal themselves at the interface between biology and nanotechnology. Indeed, we go further and assert that, as yet, we are still in the process of searching for the most suitable units to characterize the biology-nanotechnology interface.

Although our attempt to de­termine such units and scales might involve seemingly complicated inter-conversions, such as measuring distances in terms of time (via diffusion), or measuring concentrations in terms of distances, we hold that the ap­proximate numerical characterization of the scales of interest is of crucial importance to the endeavor of considering Nano mechanics at the biology-nanotechnology interface.

In particular, the right choice of units can assist us in building intuition about these systems. Our goal in this section is to emphasize the scale in length, time, force, energy, and power that are relevant in contemplating the Nano mechanics of biological systems.

Spatial Scales and Structures:

We begin with a discussion of the length scales that arise in contemplating Nano mechanics at the biology-nanotechnology interface. In this case, the prefix Nano leads justifiably to a con­sideration of the nanometer as one possible choices as the fundamental unit of length. However, to prepare ourselves for the ques­tion of how best to describe the dimensions of the spatial structures of interest here, it is important to consider the hierarchy of length scales that arise in the nabo-bio arena.

After examining this hierarchy of structures, we reformulate these length scales in terms of the volumes of these structures measure in units of the volume of a typical bacterial cell, and conclude the present section with a dis­cussion of the way that chemical concentra­tions can also be interpreted as determining a length scale.

As noted above, a first step in developing intuition concerning the spatial scales found at the biology-nanotechnology interface is through reference to the hierar­chy of scales and structures that arise in this arena. The shortest distance in this hierarchy of scales that will interest us is that associated with the size of individual atoms. We recall that the size of a hydrogen atom is roughly 0.1 nm. The scale characterizing the linkage between atoms is that of typical bond lengths that range from roughly 0.1-0.3 nm.

A step further in this hierarchy brings us to the ba­sic building blocks of the biological world such as amino acids, nucleotides, individual sugars, lipid molecules, etc. A typical length scale that characterizes these building blocks is the nanometer itself. For example, we have already made reference to the importance of lipid-bilayer membranes in bounding differ­ent region of the cell.

Phosphatideylcholine is one of the molecular building blocks of many such membranes. It has a polar head group and a hydrocarbon tail with an overall dimen­sion of 2-3 nm. Similarly, the dimensions of single amino acids and nucleotides are on the order of 1 nm as well.

As is well-known, individual nucleotides are assembled to form nucleic acids such as DNA, amino acids are assembled to form proteins, sugars combine to form polysaccharides, and lipids self-assem­ble to form membranes. One way of estimat­ing the size of the resulting molecules is by taking scale of the individual units and scal­ing up with the number of such units.

The various molecular actors of relevance to the present discussion can also be characterized by a length-scale known as the persistence length, which gives a rough description of the length over which the molecule behaves as a stiff rod. For example, the persistence length of DNA is = 50 nm, while that of the cytoskeletal filaments is in the range of 15 mm for action and 6 mm for microtubules.

Viruses as a profound example of biological nanotech­nology, the ratio of the persistence length of DNA to the size of the viral capsid (the con­tainer within which the DNA is packaged) will serve as a measure of the energetic cost of packing the DNA within the capsid and will signal the need for molecular motors to take active part in the packaging process.

The next scale above that of the various macromolecular building blocks are assemblies of such molecules in the form of various molecular machines which are some of the most com­pelling examples of nature’s nanotechnology.

The ability to begin formulating mechanistic models of such machines is founded upon key advances in both X-ray crystallography and cryo-electron microscopy. Examples of such machines and their associated dimen­sions include: the machine responsible for making the message carried by DNA readable by the protein synthesis machinery, namely, RNA polymerase (≈15 nm), the machine that produces ATP, the energy currency of the cell, namely, ATP synthase (≈10 nm), and the machine that carries out protein synthesis, namely, the ribosome (≈25 nm). A second class of assemblies of particular relevance to the present article are viruses—representative examples of which include lambda phage (≈ 27 nm), tobacco mosaic virus (≈250 nm in length), and the HIV virus (≈110-125 nm).

From the standpoint of cellular function, the next level of structural organization is as­sociated with the various organelles within the cell, structures such as the cell nucleus (3-10 pm), the mitochondria that serve as the power plant of the cell (≈1,000 nm), the Golgi apparatus wherein modifications are made to newly synthesized macromolecular components (≈1,000 nm) and so on.

These organelles should be thought of as factories in which many molecular motors of the type described earlier do their job simultaneously. At larger scales yet, and constituting a higher level of overall organization, life as nanotech­nology is revealed as cells themselves, the fundamental unit of life that is self-replicat­ing and self-sustaining.

We will make spe­cial reference to one particular bacterial cell, namely, E. coli, with typical dimensions of 1 pm. This should be contrasted with a typical eukaryotic cell such as yeast, which has linear dimensions roughly a factor of 10 larger than the E. coli cell.

We note that the various biological struc­tures described above should be seen with reference to the sorts of man-made structures to which they are interfaced. For example, optical tweezers are a means of communicating forces to macromolecules and their assemblies. Typical dimensions for the optical beads used in optical tweezers are on the scale of 500-1,000 nm. We note that though such dimensions are characteristic of organelles, they are much larger than the individual molecules they are used to study.

A second way in which individual macro­molecules are communicated with is through small tips such as those found on an AFM. In this case, the size of the order of 50 nm. Our discussion thus far has centered on the use of a single characteristic length to describe the spatial extent of biological structures. On our quest to develop intuition about the typical spiral scales found at the biology-nanotechnology interface we note that a second way to evaluate such scales is through reference to the typical volumes of the various struc­tures of interest.

As noted in the introduc­tion to this section we claim that a key way to develop intuition is by making sure to use appropriate and revealing units. For the pres­ent purpose, we argue that one useful unit of volume is that of an E. coli bacterium which, if idealized as a cylinder with diameter 1 µm and height 2 pm, has a volume of ≈ 1.5 x 10⁹ nm³.

The idea of counting the number of mol­ecules that fit into a given volume is actually quite standard. This is exactly what chemists do when they invoke the notion of concentra­tion: measuring the strength of an acid or base using pH amounts to expressing the concen­tration of H⁺ ions in solution.

Similarly, when we refer to the molarity of a given solution, it is a statement of how many copies of a given molecule will be found per liter of solution. These ideas are pertinent for other examination of the scales that arise in contemplating bio­logical nanotechnology.

As an example, we consider the action of molecular pumps such as that which maintains the concentration gradient in Ca²⁺ ions between the cellular in­terior and the extracellular medium. The Ca²⁺ pump is responsible for ensuring that the in­tercellular concentration of Ca²⁺ ions is 10-⁷ smaller than that in the cellular exterior. One explanation for this low concentration of Ca²⁺ in the cell is the role played by these ions in signaling other activities.

The low concentration might serve as a scheme for increasing the signal-to-noise ra­tio. The reported intracellular concentration of such ions is roughly 10-⁷ mm. It is inter­esting to ask how many Ca²⁺ ions this corre­sponds to in a eukaryotic cell.

The volume of a eukaryotic cell measuring 20 pm in diameter is about 4 x 10^-12 litres, which translates into ≈250 Ca²⁺ ions in the cytoplasm. For the case of E. coli, which has a volume approximately 1,000 times smaller, this concentration would correspond to one ion for every four cells. This number gives us far greater insight that the standard molar (M = mol/liter) method of expressing concentrations.

We have seen that the world of biological nanotechnology utilizes length scales that span a rather wide range, from fractions of a nanometer to tens of microns. This provides a challenge to modelling, whereby methods with atomic-scale resolution need to be com­bined in a consistent and seamless way with coarse-grained continuum descriptions to provide a complete picture. An example of such modelling will be provided in later sec­tions when we take up the mechanical aspect of the viral life cycle.

Temporal Scales and Processes:

We have seen that a study of spatial scales is a first step in our quest to understand the units that are suited to characterizing the biology- nanotechnology interface. We note that our understanding of spatial structures—both in the living world as well as those used in intriguing man-made technologies such as microelectronics—have been built around important advances such as electron micros­copy, X-ray crystallography, nuclear magnetic resonance, etc. that have revolutionized struc­tural biology and materials science alike.

On the other hand, one of the key challenges that remains in really appreciating the structure- properties paradigm—which is as central to biology as it is to materials science—is the need to acknowledge the dynamical evolu­tion of these structures.

We note that just as there is a hierarchy of spatial scales that are important to consider in describing biological nanotechnology, so, too, is there a hierarchy of temporal processes that also demand a careful consideration of rel­evant units. An impressive representation of the temporal hierarchy that must be faced in contemplating/biological systems is given by Chan and Dill.

They organize their temporal hierarchy according to factors of 1,000, start­ing at the femtosecond time scale and end­ing with time scales representative of the cell cycle itself. One of the elementary dynamical processes undergone by all of the molecular actors of the living world is thermal vibration.

Vibrations of atoms occur at the time scales of 10-¹⁵ to 10-¹² s. As will be noted later, from a modelling perspective, this depressing face manifests itself in the necessity of using time steps of the same order when integrating the equations of motion describing molecular dynamics.

The reason that this is unfortunate is that almost everything of dynamical inter­est occurs at time scales much longer that the femtosecond time scale characteristic of molecular jiggling, thus making molecular dynamics investigations computationally very expensive.

We follow Chan and Dill in examining how successive thousand fold in­creases in our temporal resolution smears out features such as vibrational motion and brings into focus more interesting processes characteristic of macromolecular function. Indeed, the side chains of amino acids ro­tate with a characteristic period on the order of 10-⁹ s.

Another thousand fold increase in time scale begins to bring key biological pro­cesses such as polymerization into view. Just as a length scale on the order of 10-100 nm is perhaps most characteristic of the length scales of biological nanotechnology, the time scale that is most relevant for considering the processes associated with biological nano­technology is something between a microsec­ond and a millisecond.

To drive home this point, we reconsider the machined described in the previous section from the length scale perspective, but now with an eye toward how fast they perform their function. Several of the molecular machines considered in the previous section mediate polymerization re­actions. For example, RNA polymerase pro­duces messenger RNA molecules by the re­peated addition of nucleotides to a chain of ever increasing length.

Such messenger RNA molecules serve in turn as the template for synthesis of new proteins by the ribosome, which reads the message contained in the RNA and adds individual amino acids onto the protein. From the temporal perspective of the present section, it is of interest to examine the rate at which new monomers are added to these polymers—roughly 10 per second in the case of RNA polymerase, and 2 per sec­ond in the case of the ribosome in eukaryotic cells (bacterial ribosomes are 10 times faster).

Once proteins are synthesized, in order to as­sume their full cellular responsibilities, they must fold into their native state—a process that occurs on time scales on the order of a millisecond. In addition to translation machines like RNA polymerase and the ribosome, there are a host of intriguing rotary machines such as ATP synthase and the flagellar rotary mo­tor. The flagellar motor, which is the means of locomotion for several bacteria, rotates at about 100 rpm.

ATP synthase makes use of proton gradient across a lipid bilayer to pro­vide rotation at a rate of roughly 6,000 rpm, approaching that of turbojet engines. Thus far, our discussion has emphasized the rate of a variety of active processes of importance to biological nanotechnology.

It is of interest to contrast these scales with those pertaining to diffusion. We note that in many instances in the biological setting the time scale of in­terest is that determined by the time it takes for diffusive communication of two spatially separated regions. In particular, the operative time scale is given by t diffusive = x²/2D

Where D is the diffusion constant and x is the distance over which the diffusion has taken place.

Force and Energy Scale: The Interplay of Deterministic and Thermal Forces:

Another set of scales of great relevance and importance to the Nano-bio interface con­cerns the nature of the forces that act in this setting. Thus far, we have examined the spa­tial extent and the cycle time of a variety of examples of biological nanotechnology. As a next step in our examination of scales, we consider the forces and energies associated with these structures. Perhaps the most com­pelling feature when thinking about forces at these scales is the interplay between deter­ministic and thermal forces.

To substantiate this claim, we note that at room temperature the fundamental energetic quantity is k_BT = 4.1 pN nm. The reason this number is of in­terest to the current endeavour is the realiza­tion that many of the molecular motors that have thus far been investigated act with forces on the pico (pN) newton scale over distances of nanometers.

The fact that the Pico newton is the relevant unit of force can be gleaned from a simple estimate. Namely, consider a typical skeletal muscle in the human arm. The cross-sec­tional size of the muscle is of order 3 cm. The muscle consists of cylindrical rods of protein called myofibrils, which are roughly 2 mm in diameter, while the myofibrils themselves are made of strands of actin and myosin fila­ments, which total some 60 nm in diameter. This gives 10¹² myosin filaments per cross-sectional area of the muscle.

As each myosin filament over the length of a single sarcomere (the contractile unit of myofibrils, some 2.5 mm long) contains some 300 myosin heads, lifting a 30 kg load corresponds to a force of 1 pN per myosin head. This is certainly an underestimate, since not all myosin heads are attached to the actin filament at the same time.

Since our estimate leaves out many de­tails, we might wonder how it compares to the measured forces exerted by molecular motors. Sophisticated experiments with opti­cal tweezers have revealed that actin-myosin motors stall at a force of around 5 pN; RNA polymerase stalls at a force of about 20 pN; the portal motor of the f-29 bacteriophage exerts forces up to 50 pN.

RNA polymerase is a molecular motor that moves along DNA while transcribing genes into messenger RNA. The DNA itself is an elastic object that is that of an entropic spring with a stretch modulus of 0.1 pN, while, at forces exceeding 10 pN, its stretch modulus is determined by hydrogen bonding of the base pairs and is roughly 1,000 pN. All the above- mentioned data reinforces the argument, that Pico newton is the relevant unit of force in the Nano mechanical world of the cell.

The concept of ‘stress’ is closely related to that of force. Stress is a continuum mechani­cal concept of force per unit area, and it has been used with success in solid and fluid me­chanics. We extend the idea of stress to the Nano mechanical level to see what numbers we arrive at.

From the data above we can deduce that a single myosin fiber sustains a stress of about 10~² pN/nm², which is the same as 10^-2 MPa. Migratory animal cells such as fibroblasts—which are responsible for scav­enging and destroying undesirable products in tissues—can generate a maximum stress on their substrate on the order of 3.0 x 10^-2 MPa. DNA can sustain stresses in the excess of 20 pN/nm², or 20 MPa, at which point an interesting structural transition accompanied by an overall increase of its contour length is observed.

Engineering materials such as steel and aluminium, on the other hand, can sus­tain stresses of about 100 MPa. This goes to show that nanotechnology in the context of biological systems is built from rather soft materials. It is of interest to translate our intuition concerning Pico newton forces into corre­sponding energetic terms. The kinesin motor advances 8 nm in each step against forces as high as 5 pN. This translates to a work done on the order of 40 pN nm.

A myosin motor suf­fers displacements of about 15 nm with forces in the Pico newton range. ATP hydrolysis (to ADP) releases energy (at pH 7 and room tem­perature) of about 50 pN nm. When a titin molecule is pulled, it unfolds under forces of 30-300 pN, causing discrete expansions of 10-30 nm, implying energies in the range of 300-9,000 pN nm.

Experimental data provide a compelling argument in favour of thinking of the pN nm as a unit of energy for Nano mechanics. However, the observation that k_BT = 4.1 pN nm at room temperature gives important insight—since it reveals that thermal forces and entropic effects—play a competing role in biological Nano mechanics. This pro­vides nature with unique design challenges, whereby molecular motors that can perform useful work must do so in the presence of strong thermal fluctuations for the normal functioning of the cell.

Operation of motors in such a noisy envi­ronment is governed by laws that are proba­bilistic in nature. This is manifest in single molecule experiments that observe motors stalling and sometimes reversing direction. Energy conversion is crucial to any develop­mental or evolutionary process. The steam engine powered the industrial revolution. However, long before thinking begins with man-made machines which founded the in­dustrial revolution, power generation had already become a central part of life’s nanotechnology.

Indeed, the development of ATP synthase is one of the cornerstones for the evolution of higher life-forms. An inevitable concomitant of evolution is the necessity for faster and more efficient operations. The in­dustrial revolution led to the emergence of complex and intelligent organisms. Invari­ably, a machine or an organism is limited in its abilities by the speed at which it can con­vert one form of energy (usually chemical) to other forms of energy (usually mechanical). This is why a study of their ‘power plants’ be­comes important.

Power plants (or engines) are usually characterized by their force-veloc­ity curves and compared using their power- to-weight (P/W) ratio. For example, the myo­sin motor has a P/W ratio of 2 x 10⁴ W/kg, the bacterial flagellar motor stands at 100 W/ kg, an internal combustion engine is at about 300 W/kg, and a turbojet engine stands at 3,000 W/kg. These figures tell us that linear motors like myosin and kinesin are extremely powerful machines.

Modelling at the Nano-Bio Interface:

We have already provided a number of dif­ferent views of the biology-nanotechnology interface, all of which reveal the insights that can emerge from model building. Indeed, one of the key thrusts of this entire article is the view—as the type of data that emerges con­cerning biological systems becomes increas­ingly quantitative, it must be responded to with models that are also quantitative.

The plan of this section is to show how atomistic and continuum analyses each offer insights into problems of Nano technological signifi­cance, but, under some circumstances, both are found wanting and it is only through a synthesis of both types of models that certain problems will surrender. The plan of this sec­tion is to examine the advantages and difficul­ties associated with adopting both atomistic and continuum perspectives and then to hint at the benefits of seeking mixed representa­tions.

University and Specificity:

One of the key insights concerning model building in Nano mechanics, whether we are talking about the Nano scale tri-biological ques­tions pertinent to magnetic recording or the operations of molecular motors, is that such questions live in the no-man’s-land between traditional continuum analysis at one ex­treme and all-atom approaches such as mo­lecular dynamics on the other.

Indeed, there is much discussion about the breakdown of continuum mechanics in modelling the me­chanics of systems at the Nano scale. This dichotomy between continuum theories, which treat matter as continuously distrib­uted, and atomic-level models, which explic­itly acknowledge the graininess of matter, can be restated in a different (and perhaps more enlightening) way.

In particular, it is possible to see atomistic and continuum theories as offering complementary views of the same underlying physics. Continuum models are suitable for characterizing those features of’ a system that can be thought of as averages over the underlying microscopic fluctuations. By way of contrast, atomistic models reveal the details that a continuum model will never capture, and, in particular, they shed light on the specificity of the problem at hand.

The perspective adopted here is that con­tinuum models and atomistic models each reveal important features of a given problem. For example, in contemplating the competition between fracture and plasticity at crack tips, a continuum analysis provides critical insights into the nature of the elastic fields surrounding a defect such as a crack.

These fields adopt fundamental and universal form at large scales with all detailed mate­rial features buried in simple material param­eters. By way of contrast, the precise details of the dissipative processes occurring at a crack tip (in particular, the competition between bond breaking with the creation of new free surface and dislocation nucleation) require detailed atomic-level descriptions of the en­ergetics of bond stretching and breaking.

In the biological setting, similar remarks can be made. In certain instances, the description of biological polymers as random coils suffices and yields insights into features such as the mean size of the polymer chain as a func­tion of its length. On the other hand, if our objective is mechanistic understanding of processes such as how phosphorylation of a particular protein induces conformational change, this is an intrinsically atomic-level question.

The language we invoke to describe this dichotomy is the use of the terms uni­versality and specificity, where, as described above, insights of a universal character refer to those features of systems that are generic, while specificity refers to the features of sys­tems that depend upon precise details such as whether or not a particular molecule is bound at a particular site.

Atomic-Level Analysis of Biological System:

One of the intriguing roles of nanotechnology in the biological setting is that it has brought the Intron technology of traditional solid me­chanics to the Nano scale and has permitted the investigation of the force-extension char­acteristics of Nano scale systems (macro-molecules and their assemblies, in particular). Mechanical force spectroscopy is emerging as a profound tool for exploring the connection between structure, force and chemistry, in much the same way that conventional stress-strain tests provided insights into the connection between structure and properties of conventional materials.

The ob­jective of this section is to call the reader’s at­tention to the types of modelling that can be done from an all atom perspective. What ex­actly is meant by a model in this setting? We begin by noting that for the purposes of the general discussion given here; the same basic ideas are present whether one is modelling tri biological processes such as the sliding of adjacent surfaces, or attempting to examine the operation of a protein machine such as ATP synthase.

The set of degrees of freedom considered by the atomic-scale modeller is the full set of atomic positions (R₁ R₂,… R_N), which we also refer to as {R_i}. For most purposes, one proceeds through reference to a classical potential energy function, E_tot ({R_i}), which is a rule that assigns energy for every configuration {R_i}. While it would be most appealing to be able to perform a full quantum mechanical analysis, such calcula­tions are computationally prohibitive. Given the potential energy, the forces on each and every atom can be computed where, for ex­ample, the force in the (α^th Cartesian direction on the i^th atom is given by

For those interested in finding the energy minimizers, such forces can be used in conjunction with methods such as the conjugate gradient method or the Newton-Raphson method. Alternatively, many questions are of a dynamic character, and, in these cases, Newton’s equations of motion are integrated, thus permitting an investigation of the tem­poral evolution of the system of interest.

We further note that in the case of titin there has been an especially pleasing syn­ergy between the atomic-scale calculations and the corresponding force-extension mea­surements. In particular, in response to the suggestion that it was a particular set of hy­drogen bonds that impugned in the rupture process, mutated versions of the titin protein were created in which the number of such hydrogen bonds was changed with the result that the rupture force was changed according to expectation. This can be seen as a primitive example of the ultimate goal of tailoring new materials (both biological and otherwise) through appropriate computer modelling.

Continuum Analysis of Biological Systems:

We have already noted that there are many appealing features to strictly continuum anal­yses of material systems. In particular, models based upon continuum mechanics result in a mathematical formulation that permits us to uncork the traditional tools associated with partial differential equations and functional analysis.

In keeping with our argument that it is the role of models to serve our intuition, the ability to write down continuum models raises the possibility of obtaining analytic so­lutions to problems of interest, an eventual­ity that is nearly impossible once the all-atom framework has been adopted.

Macromolecules as Elastic Rods:

Whether we contemplate the information carrying nu­clei acids, the workhorse proteins, or energy storing sugars, the molecular business of the living world is dominated by long chain mol­ecules. For the model builder, such molecules suggest two complementary perspectives, each of which contains a part of the whole truth. On the one hand, polymer physicists have gained huge insights by thinking of long chain molecules as random-walks.

Stated simply, the key virtue of the random-walk description of long chain molecules is that it reflects the overwhelming importance of en­tropy in governing the geometric conforma­tions that can be adopted by macromolecules. The other side of the same coin considers long chain molecules as elastic rods with stiffness that governs their propensity for bending. We will take up this perspective in great detail in the final section of this article when we con­sider the energetics of DNA packaging in viruses. For our present purposes, we examine one case study in treating macromolecules as elastic rods.

The example of interest here is chosen in part because it reflects another fascinating aspect of biological nanotechnol­ogy, namely, regulation and control. It is well-known that genes are switched on and off as they are needed.

The basic idea can be eluci­dated through the example of a particular set of genes in E. coli: the lac operon. There are a set of enzymes exploited by this bacterial cell when it needs to digest the sugar lactose. The gene that codes for these enzymes is only turned on when lactose is present and certain other sugars are absent.

The Nano technological solution to the problem of regulating this gene has been solved by nature through the presence of a molecule known as the lac re­pressor, which binds onto the DNA in the region of this lac gene and prevents the gene from being expressed. More specifically, the lac repressor binds onto two different regions of the DNA molecule simultaneously, form­ing a loop in the intervening region, and thus rendering that region inoperative for tran­scription.

We have belabored the difficulties that attend the all-atom simulation of most problems of Nano technological interest— system sizes are too small and simulated times are too short. On the other hand, it is clearly of great interest to probe the atomic level dynamics of the way that molecules such as lac repressor interact with DNA and there­by serve as gene regulators.

Membranes as Elastic Media:

One way to classify biological structures is along the lines of their dimensionality. We examined the sense in which many of the key macromolecules of the living world can be thought of as one-dimensional rods. The next level in this dimensional hier­archy is to consider the various membranes that compartmentalize the cell and which can be examined from the perspective of two-dimensionality. In the previous discus­sion, we examined the sense in which many of the key macromolecules of the living world can be thought of as one-dimensional rods.

The next level in this dimensional hierarchy is to consider the various membranes that compartmentalize the cell and which can be examined from the perspective of two-dimensional elasticity. Just as there are a huge number of modelling questions to be posed concerning the structure and function of long chain molecules such as nucleic acids and proteins, there is a similar list of questions that attend the presence of a host of differ­ent membranes throughout the cell.

To name a few, we first remind the reader that such membranes are full of various proteins that serve in a variety of different capacities, some of which depend upon the mechanical state of the membrane itself. In a different vein, there has been great interest in examining the fac­tors that give rise to the equilibrium shapes of cells. In both instances, the logic associ­ated with building models of these phenom­ena centres on the construction of an elastic Hamiltonian that captures the energetics of deformations expressed in terms of surface area, curvature, and variations in thickness.

Also, just as there has been great progress in measuring the properties of single proteins and nuclei acids, there has been considerable progress in examining the force response of membranes as well. The prospect of bringing the tools of traditional continuum theory to bear on problems of biological significance is indeed a daunting one.

As noted earlier, to our way of thinking, one of the biggest chal­lenges posed by biological problems is the dependence of these systems on detailed mo­lecular structures and dynamics—what we have earlier characterized as specificity’, one of the hallmarks of biological action.

Nature’s Nanotechnology: Viruses as an Example:

In this article, we have pre­sented different facets of the relationship be­tween biology and nanotechnology and the modelling challenges they encompass. For example, we have noted that two key aspects of nature’s nanotechnologies are the exploita­tion of self-assembly processes for the con­struction of molecular machines and the role of active processes mediated by molecular motors. We have also argued for the syner­gistic role of nanotechnology in producing new methods for the experimental analysis of biological systems with examples ranging from new molecular dyes to the use of optical tweezers.

Finally, we have described some of the modelling challenges posed by contem­plating the biology-nanotechnology interface and the sorts of coarse-grained models that have arisen to meet these challenges. In this final section, we present a discussion of virus­es as a case study at the confluence of these different themes. Though the importance of viruses from a health perspective is well-known even to the casual observer, they are similarly impor­tant both from the technological perspective and as a compelling and profound example of nature’s nanotechnology par excellence.

To appreciate the sense in which viruses serve as a compelling example of biological nano­technology, we begin by reviewing the nature of the viral life cycle with special reference to the case of bacterial viruses (i.e., viruses that infect bacteria), known as bacteriophages. For concreteness, we consider the life cycle of a bacteriophage such as the famed lamb­da phage that infects E. coli. The life cycle of such viruses is shown schematically in (Fig. 4.3).

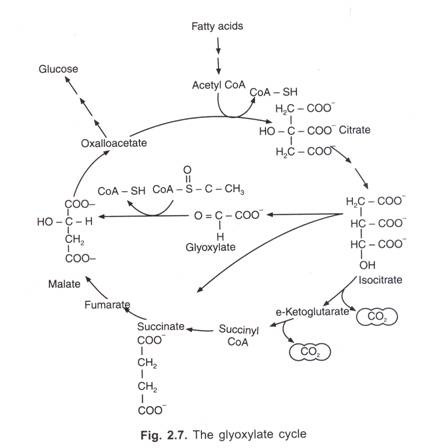Upon an encounter with the E. coli host, the virus attaches to a receptor (protein) em­bedded in the bacterial membrane and ejects its DNA into the host cell, leaving an empty capsid as refuse from the process. As an aside, it is worth nothing that experiments such as the famed Hershey-Chase experiment used tagged DNA and tagged proteins on viruses to settle the question of whether proteins or nuclei acids are the carriers of genetic infor­mation.

The outcome of these experiments was the conclusion that DNA is the genetic material. For the purposes of the present dis­cussion, the other interesting outcome of the Hershey-Chase experiment is that it provides insights into the mechanistic process associ­ated with delivery of the viral genome.

Once the viral genome has been delivered to the host cell (we now oversimplify), the replica­tion machinery E. coli is hijacked to do the virus’ bidding. In particular, the genes coded for in the viral DNA are expressed, and the proteins needed to make copies of the phage particle (i.e., the ingredients for an eventual self-assembly process) are created.

Interestingly, part of the gene products as­sociated with this process are the components of the molecular motor, which is responsible for packaging the replicated viral DNA into the new protein capsids that will eventually become the next generation of viruses. In­deed, once assembled, this motor takes the replicated viral DNA and packs it into the viral capsid. Recall our insistence that an­other of the important themes presented by biological examples of nanotechnology is the huge role played by active processes that re­flect Mechano-chemical coupling.

Once the packaging process is completed, and the re­maining parts of the self-assembly process have been effected (such as the attachment of the viral sheath and legs whereby the phage attaches to host cells), enzymes are released that breakdown the cell wall of the infected cell with the ultimate result that what started as a single bacteriophage has, in less than an hour, become on the order of 100 new phage particles ready to infect new cells.

A second sense in which viruses are deserving of case study status in this article is the role viruses—such as lambda phage—play in biotechnology it­self. For example, from the standpoint of both cloning and the construction of genomic li­braries, the use of viruses is commonplace. From a more speculative perspective, viruses are also drawing increasing attention from the standpoint of gene therapy (as a way of delivering DNA to specific locations) and, more generally, as small scale containers.

To substantiate these assertions, we briefly con­sider the construction of genomic libraries and the role played by viruses in these ma­nipulations. We pose the following question: Given that the length of the human genome is on the order of 10⁹ base pairs, how can one organize and store all of this genetic informa­tion for the purposes of experiments such as the sequencing of the human genome? One answer to this challenge is the use of bacteriophage to deliver phage DNA to E. coli cells, but with subtlety that the delivered DNA fragments have ligated within them fragments of the human genome whose lengths are of the order of 10 kb (kb = kilo base-pairs).

The particulars of this procedure involve first cut­ting the DNA into fragments of roughly 10 kb in length using a class of enzymes known as restriction enzymes. The result of this op­eration is that the original genome is now separated into a random collection of frag­ments.

These fragments are then mixed with the original lambda phage DNA that has also been cut at a single site such that the ge­nomic fragments and the lambda fragments are complementary. Using a second enzyme known as ligase, the genomic DNA is joined to the original lambda phage fragments so that the resulting DNA resembles the original lambda DNA, but now with a 10 kb fragment inserted in the middle. These cloned lambda DNA molecules are then packaged into the lambda phage using a packaging. The result­ing lambda phage, now fully packed with cloned DNA, are used to infect E. coli cells, and the cloned DNA, once in the bacterial cell, circularizes into a DNA fragment known as a plasmid.

The plasmid is then passed from one generation of E. coli to the next. This is the so called pro-phage pathway in which, unlike the lytic pathway shown in Fig. 4.3, the bacterio­phage is latent and does not destroy the cell. Note that different E. coli cells are infected with viruses containing different cloned frag­ments.

As a result, the collection of all such E. coli cells constitutes a library of the original genomic DNA. We round out our introduc­tory discussion on viruses with discussion of the compelling recent experiments that have been performed to investigate the problem of viral packing and, similarly, how ideas like those described in this article can be used to model these processes.

Scientists have used optical tweezers to measure the force applied by the packaging motor of the ●-29 bacterio­phage during the DNA packaging process. In particular, they measured the force and rate of packaging as a function of the amount of DNA packed into the viral head with the re­sult that as more DNA is packed, the resistive force due to the packed DNA increases and the packing rate is reduced.

As noted above, the viral problem is inter­esting not only because it exemplifiers many of the features of biological nanotechnology introduced throughout this article, but also illustrates the way in which model building has arisen in response to experimental in­sights. The various competing energies that are implicated in the DNA packing process have been described by Riemer and Bloom- field.

The energetics of viral packing is char­acterized by a number of different factors, including:

(i) The entropic-spring effect that causes the DNA in solution to adopt a more spread out configuration than that in the viral capsid,

(ii) The energetic of elastic bending, which results from inducing curvature in the DNA on a scale that is considerably smaller than the persistence length of ∑p ≈ 50 nm, and

(iii) Those factors related to the presence of charge both on the DNA itself and in the surrounding solution.

As shown by Riemer and Bloomfield, the entropic contribution is smaller by a factor of 10 or more relative to the bending energies and those mediated by the charges on the DNA and the surrounding solution, and, hence, we make no further reference to it.

As a result, just like in earlier work, we examine the interplay of elastic and interaction forces, though we neglect sur­face terms originating from DNA-capsid and DNA-solvent interactions. We note that the viral packing process involves DNA segments with lengths on the order of 10 pm and takes place on the time scale of minutes.

As a result, from a modelling perspective, it is clear that such problems are clearly out of reach of con­ventional molecular dynamics. As a result, a continuum description of the DNA packing process with the proviso that such models will ultimately need to be refined to account for the sequence dependence of the elasticity of DNA are being carried out.

Concluding Remarks:

One of the most compelling areas to be touched by nanotechnology is biological sci­ence. Indeed, we have argued that there is a fascinating interplay between these two sub­jects, with biology as a key beneficiary of ad­vances in nanotechnology as a result of a new generation of single molecule experiments that complement traditional assays involv­ing statistical assemblages of molecules.

This interplay runs in both directions with nano­technology continually receiving inspiration from biology itself. The goal of this article has been to highlight some representative ex­amples of the interplay between biology and nanotechnology and to illustrate the role of Nano mechanics in this field and how me­chanical models have arisen in response to the emergence of this new field.

Primary attention has been given to the particular example of the processes that attend the life cycle of bac­terial viruses. Viruses feature many of the key lessons of biological nanotechnology, includ­ing self-assembly, as evidenced in the sponta­neous formation of the protein shell (capsid) within which the viral genome is packaged and a motor-mediated biological process, namely, the packaging of DNA in this capsid by a molecular motor that pushes the DNA into the capsid.

We argue that these processes in viruses are a compelling real-world exam­ple of nature’s nanotechnology and reveal the Nano mechanical challenges that will continue to be confronted at the nanotechnology-biology interface. This article advances the view that biological nanotechnology serves as an inspiring vision of what nanotechnology can offer. Moreover, developing simple models of nature’s nanotechnology can provide impor­tant insights into viable strategies for making machines at the Nano scale.

We have argued that the advance of single molecule technologies presents both scien­tific and technological possibilities. Both classes of questions imply significant model­ling demand, just as earlier advances in other settings such as in materials for microelec­tronics applications, did.

We claim that these modelling challenges are perhaps more acute as a result of the vicious chemical and struc­tural non-homogeneity of biological systems. As a result, we see an ever-increasing role for modelling methods that aim to keep atomic level specify where needed, while rejecting such resolution elsewhere.